Circular Basin

UNDER CONSTRUCTION

Analytical Solution

Dupont (2001) presented an analytical solution for a closed circular domain on an f-plane, with radius $R$, a linear bottom friction, and a spatially variable wind stress equal to $\tau _{Wx}=Wy/R$, $\tau _{Wy}=0$ where $W$ is the gradient of the wind forcing and $y$ is the vertical coordinate. The water surface elevation solution is given by

$\eta ={\begin{cases}{\frac {W{{r}^{2}}\sin 2\theta }{4gHR}},&{\mbox{if }}f_{c}=0\\{\frac {W{{f}_{c}}}{RgH\kappa }}\left[{\frac {{R}^{2}}{8}}+{\frac {{r}^{2}}{4}}\left({\frac {\kappa }{{f}_{c}}}\sin 2\theta -1\right)\right],&{\mbox{if }}f_{c}\neq 0\\\end{cases}}$

(1)

The current velocities are independent of the Coriolis parameter and are given by

Failed to parse (SVG with PNG fallback (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://en.wikipedia.org/api/rest_v1/":): {\displaystyle u = \frac{W y }{2R\kappa }

(2)

Failed to parse (syntax error): {\displaystyle v = -\frac{W x }{2R\kappa }

(3)

Setup

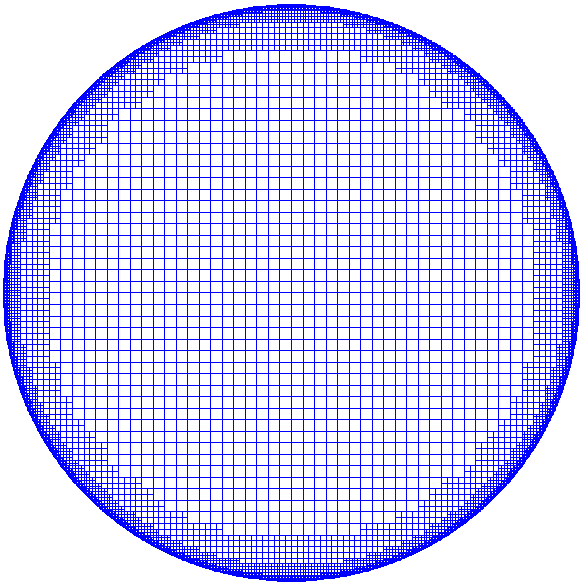
The model is run to steady state from zero current and water level initial conditions with , , and both , and . Figure 1 shows the computational grid with 5 levels of refinement from 2000 m to 125 m.

Figure 1. Computational grid.

Results

Table 2. Goodness of fit statistics for the water elevation

Statistic	Value
RMSE	0.0074 m
RMAE	0.0068
R^2	0.991
Bias	0.0017 m

References

• Dupont, F., 2001. Comparison of numerical methods for modelling ocean circulation in basins with irregular coasts. Ph.D. thesis, McGill University, Montreal.

---

Test Cases

Documentation Portal