Long wave propagation: Difference between revisions

From CIRPwiki
Jump to navigation Jump to search
(Created page with '== '''Setup''' == Forcing: 0.3048 m amplitude M2 tide (red cell string) Bottom friction: 0 sec/m^(1/3) (Manning N) Horizontal eddy viscosity: 0 m^2/sec Run duration: 5 days …')
 
 
(29 intermediate revisions by the same user not shown)
Line 1: Line 1:
== '''Setup''' ==
= Problem =
Lynch and Gray (1978) presented the analytical solution for depth-averaged long-wave propagation in am annular domain. Here the case tested with a linearly sloping bed, and without bottom friction, Coriolis, or horizontal mixing. The offshore boundary consists of an single tidal constituent. Table 1 summarizes the important model settings used for this test.


Forcing: 0.3048 m amplitude M2 tide (red cell string)
'''Table 1. General Settings for quarter annulus test'''
{|border="1"
|'''Parameter''' ||'''Value'''
|-
|Deepwater tidal amplitude|| 0.3048 m
|-
|Tidal period || 12.42 hrs (M2)
|-
|Inner radius || 60.96 km
|-
|Outer radius || 152.4 km
|-
|Depth at inner radius || 10.02 m
|-
|Depth at outher || 25.05 m
|-
|Bottom  friction || None
|-
|Horizontal eddy viscosity || 0.0
|-
|Corilis || 0.0
|}


Bottom friction: 0 sec/m^(1/3) (Manning N)
= Model Setup =
[[Image:QA_Depth_8km.png|thumb|right|600px| Figure 1. Quarter Annulus]]
The computational domain (Figure 1) consists of a three-level telescoping Cartesian grid with a grid resolution of 4, 2, and 1 km for each level. Higher resolution is specified near the inner and outer boundaries in order to reduce errors associated with the representation of the curved boundaries with squares. The computational grid is shown in Figure 1. The grid has 1,160 active ocean cells. The important model settings are shown in Table 2.


Horizontal eddy viscosity: 0 m^2/sec
'''Table 2. General settings for the quarter annulus test'''
{|border="1"
|'''Parameter''' ||'''Value'''
|-
| Solution scheme || Implicit
|-
|Time step || 600 sec (10 min)
|-
|Simulation duration || 120 hrs
|-
|Ramp period  || 24 hrs
|-
|Advection terms || Off
|-
|Mixing terms || Off
|-
|Wall friction || Off
|-
|Coriolis || Off
|}


Run duration: 5 days
<br style="clear:both" />


Ramp period: 2 days
= Results =
[[Image:QA_Water_Level_8km.png|thumb|right|600px| Figure 2. Comparison of analytical (solid black) and calculated (red dots) water surface elevation at the center of the inner radius. ]]
Figure 2 shows a time series of water levels at the inner edge of the model domain. The goodness of fit statistics are listed in Table 3. The model accurately predicts the wave phase but slightly overestimates the amplitude by approximately 0.01 m. No significant numerical dissipation is observed or numerical instabilities. The simulation takes about 52 seconds on a PC and a single processor.


Time step: 10 min
'''Table 3. Goodness of fit statistics for the water elevation'''
{|border="1"
|'''Statistic''' ||'''Value'''
|-
|NRMSE || 3.3 %
|-
|NRMAE || 2.7 %
|-
|R^2  || 0.999
|-
|Bias || 0.002 m
|}


Wall Friction: Off
Grid resolution: Constant 1000 x 1000 m
Number of cells: 23409
[[Image:Quarter_annulus_grid.PNG|thumb|left|400px|Quarter Annulus | alt=Quarter Annulus]]
<br style="clear:both" />
<br style="clear:both" />


== '''Results''' ==
= References =
 
* Lynch, D.R., and Gray, W.G. (1978). "Analytical solutions for computer flow model testing," J. Hydraulics Division, 104, 1409-28.
The figure below shows a time series of water
 
levels at the green point shown in the figure above.
 
[[Image:Quarter_Annulus_TimeSeries.PNG|thumb|left|800px|Results | alt=Results]]
<br style="clear:both" />


----
----
[[Test_Cases]]
[[Test_Cases]]

Latest revision as of 23:15, 1 June 2011

Problem

Lynch and Gray (1978) presented the analytical solution for depth-averaged long-wave propagation in am annular domain. Here the case tested with a linearly sloping bed, and without bottom friction, Coriolis, or horizontal mixing. The offshore boundary consists of an single tidal constituent. Table 1 summarizes the important model settings used for this test.

Table 1. General Settings for quarter annulus test

Parameter Value
Deepwater tidal amplitude 0.3048 m
Tidal period 12.42 hrs (M2)
Inner radius 60.96 km
Outer radius 152.4 km
Depth at inner radius 10.02 m
Depth at outher 25.05 m
Bottom friction None
Horizontal eddy viscosity 0.0
Corilis 0.0

Model Setup

Figure 1. Quarter Annulus

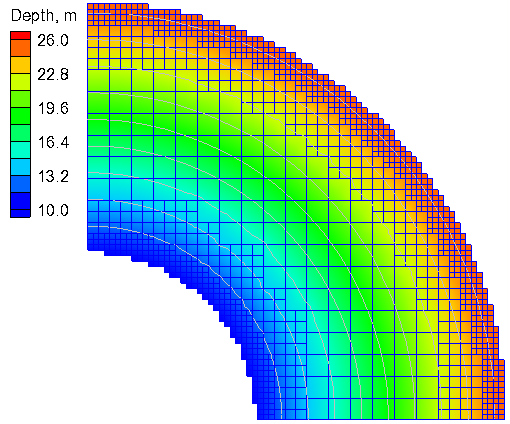
The computational domain (Figure 1) consists of a three-level telescoping Cartesian grid with a grid resolution of 4, 2, and 1 km for each level. Higher resolution is specified near the inner and outer boundaries in order to reduce errors associated with the representation of the curved boundaries with squares. The computational grid is shown in Figure 1. The grid has 1,160 active ocean cells. The important model settings are shown in Table 2.

Table 2. General settings for the quarter annulus test

Parameter Value
Solution scheme Implicit
Time step 600 sec (10 min)
Simulation duration 120 hrs
Ramp period 24 hrs
Advection terms Off
Mixing terms Off
Wall friction Off
Coriolis Off


Results

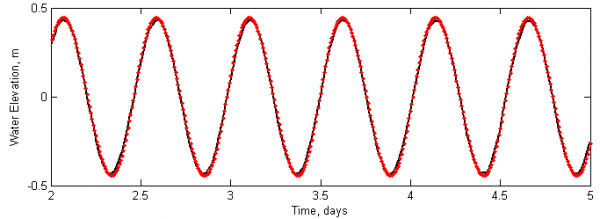
Figure 2. Comparison of analytical (solid black) and calculated (red dots) water surface elevation at the center of the inner radius.

Figure 2 shows a time series of water levels at the inner edge of the model domain. The goodness of fit statistics are listed in Table 3. The model accurately predicts the wave phase but slightly overestimates the amplitude by approximately 0.01 m. No significant numerical dissipation is observed or numerical instabilities. The simulation takes about 52 seconds on a PC and a single processor.

Table 3. Goodness of fit statistics for the water elevation

Statistic Value
NRMSE 3.3 %
NRMAE 2.7 %
R^2 0.999
Bias 0.002 m


References

  • Lynch, D.R., and Gray, W.G. (1978). "Analytical solutions for computer flow model testing," J. Hydraulics Division, 104, 1409-28.

Test_Cases

Retrieved from "https://cirpwiki.info/index.php?title=Long_wave_propagation&oldid=6932"