Clear water jet: Difference between revisions
Created page with '== '''Setup''' == Normal 0 false false false MicrosoftInternetExplorer4 The rectangular flume has a narrow 0.2 m-wide inlet and a 3 m-wide outlet.…' |
No edit summary |
||
| Line 1: | Line 1: | ||
== '''Setup''' == | == '''Setup''' == | ||
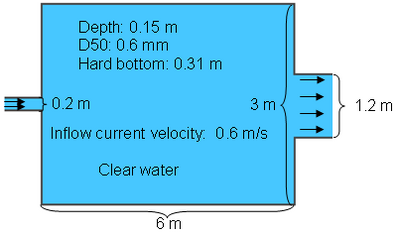
The rectangular flume has a narrow 0.2 m-wide inlet and a 3 m-wide outlet. The initial water depth was 0.15 m with a 0.16 m layer of 0.6 mm sand over a concrete bottom. The flume was 5 m long and 4 m wide. The measured sand settling velocity of 0.013 m/s was used. | |||
Normal 0 false false false MicrosoftInternetExplorer4 | Normal 0 false false false MicrosoftInternetExplorer4 | ||
The | The computational mesh consisted of 62 rows and 69 columns (Figure 2). The computational time was 30 seconds. The transport equation which best fit the measurements was the Soulsby-Van Rijn (1993). The bed and suspended load transport scaling factors were calibrated to 2.0 which is within the acceptable range for the transport formula scatter. | ||
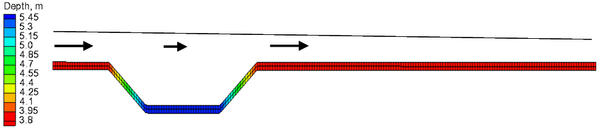
The experiment consist of a trapezoidal channel of uniform find sand (d50 = 0.16 mm) with a steady flow (0.51 m/sec) perpendicular to the channel axis (Gallappatti and Vreugdenhil 1985). The water depth on the upstream bank is about 0.39 m. Three bank slopes were tested: 1:3, 1:7, and 1:10. A flux boundary condition was used at the inlet and a water level boundary condition at the outlet. A constant Manning n coefficient of 0.025 (default) was used. The Lund-CIRP transport formula was used for all three test cases. The morphologic scaling factor was set to 1.0. The adaptation length was calibrated to be 0.6 m. | The experiment consist of a trapezoidal channel of uniform find sand (d50 = 0.16 mm) with a steady flow (0.51 m/sec) perpendicular to the channel axis (Gallappatti and Vreugdenhil 1985). The water depth on the upstream bank is about 0.39 m. Three bank slopes were tested: 1:3, 1:7, and 1:10. A flux boundary condition was used at the inlet and a water level boundary condition at the outlet. A constant Manning n coefficient of 0.025 (default) was used. The Lund-CIRP transport formula was used for all three test cases. The morphologic scaling factor was set to 1.0. The adaptation length was calibrated to be 0.6 m. | ||
| Line 11: | Line 13: | ||
== '''Results''' == | == '''Results''' == | ||
The figures below show a comparison between measured and computed bed elevations. | The figures below show a comparison between measured and computed bed elevations. | ||
| Line 24: | Line 25: | ||
== '''References''' == | == '''References''' == | ||
Thuc, T. (1991) “Two-dimensional morphological computations near hydraulic structures”, Doctoral Dissertation, Asian Institute of Technology, Bangkok, Thailand. | |||
---- | ---- | ||
[[Test_Cases]] | [[Test_Cases]] | ||
Revision as of 17:17, 26 August 2010
Setup
The rectangular flume has a narrow 0.2 m-wide inlet and a 3 m-wide outlet. The initial water depth was 0.15 m with a 0.16 m layer of 0.6 mm sand over a concrete bottom. The flume was 5 m long and 4 m wide. The measured sand settling velocity of 0.013 m/s was used.
Normal 0 false false false MicrosoftInternetExplorer4
The computational mesh consisted of 62 rows and 69 columns (Figure 2). The computational time was 30 seconds. The transport equation which best fit the measurements was the Soulsby-Van Rijn (1993). The bed and suspended load transport scaling factors were calibrated to 2.0 which is within the acceptable range for the transport formula scatter.
The experiment consist of a trapezoidal channel of uniform find sand (d50 = 0.16 mm) with a steady flow (0.51 m/sec) perpendicular to the channel axis (Gallappatti and Vreugdenhil 1985). The water depth on the upstream bank is about 0.39 m. Three bank slopes were tested: 1:3, 1:7, and 1:10. A flux boundary condition was used at the inlet and a water level boundary condition at the outlet. A constant Manning n coefficient of 0.025 (default) was used. The Lund-CIRP transport formula was used for all three test cases. The morphologic scaling factor was set to 1.0. The adaptation length was calibrated to be 0.6 m.
Results
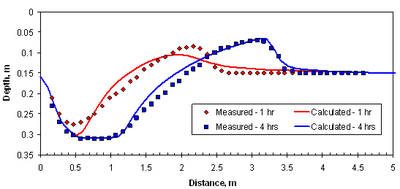
The figures below show a comparison between measured and computed bed elevations.

References
Thuc, T. (1991) “Two-dimensional morphological computations near hydraulic structures”, Doctoral Dissertation, Asian Institute of Technology, Bangkok, Thailand.