Circular Basin: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| Line 1: | Line 1: | ||
__NOTOC__ | __NOTOC__ | ||
<font color=red>'''UNDER CONSTRUCTION'''</font> | <font color=red>'''UNDER CONSTRUCTION'''</font> | ||
=Analytical Solution= | =Analytical Solution= | ||
Dupont (2001) presented an analytical solution for a closed circular domain on an f-plane, with radius , a linear bottom friction, and a spatially variable wind stress equal to , where is the gradient of the wind forcing and is the vertical coordinate. The water surface elevation solution is given by | Dupont (2001) presented an analytical solution for a closed circular domain on an f-plane, with radius , a linear bottom friction, and a spatially variable wind stress equal to , where is the gradient of the wind forcing and is the vertical coordinate. The water surface elevation solution is given by | ||
{{Equation| <math> | |||
\eta =\left\{ \begin{align} | |||
</math> |2=1}} | |||
= Setup = | = Setup = | ||
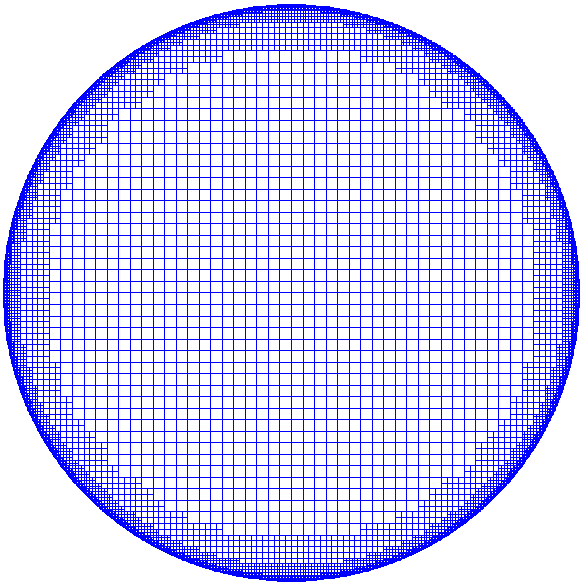
The model is run to steady state from zero current and water level initial conditions with , , and both , and . Figure 1 shows the computational grid with 5 levels of refinement from 2000 m to 125 m. | The model is run to steady state from zero current and water level initial conditions with , , and both , and . Figure 1 shows the computational grid with 5 levels of refinement from 2000 m to 125 m. | ||
[[Image:Grid_CB3.png|thumb|right|600px| Figure 1. Computational grid.]] | |||
<br style="clear:both" /> | <br style="clear:both" /> | ||
= Results = | |||
Table 2. Goodness of fit statistics for the water elevation | |||
{|border="1" | |||
|'''Statistic''' ||'''Value''' | |||
|- | |||
|RMSE || 0.0074 m | |||
|- | |||
|RMAE || 0.0068 | |||
|- | |||
|R^2 || 0.991 | |||
|- | |||
|Bias || 0.0017 m | |||
|} | |||
== References == | == References == | ||
* Dupont, F., 2001. Comparison of numerical methods for modelling ocean circulation in basins with irregular coasts. Ph.D. thesis, McGill University, Montreal. | * Dupont, F., 2001. Comparison of numerical methods for modelling ocean circulation in basins with irregular coasts. Ph.D. thesis, McGill University, Montreal. | ||
Revision as of 22:43, 11 May 2011
UNDER CONSTRUCTION
Analytical Solution
Dupont (2001) presented an analytical solution for a closed circular domain on an f-plane, with radius , a linear bottom friction, and a spatially variable wind stress equal to , where is the gradient of the wind forcing and is the vertical coordinate. The water surface elevation solution is given by
| Failed to parse (unknown function "\begin{align}"): {\displaystyle \eta =\left\{ \begin{align} } | (1) |
Setup
The model is run to steady state from zero current and water level initial conditions with , , and both , and . Figure 1 shows the computational grid with 5 levels of refinement from 2000 m to 125 m.
Results
Table 2. Goodness of fit statistics for the water elevation
| Statistic | Value |
| RMSE | 0.0074 m |
| RMAE | 0.0068 |
| R^2 | 0.991 |
| Bias | 0.0017 m |
References
- Dupont, F., 2001. Comparison of numerical methods for modelling ocean circulation in basins with irregular coasts. Ph.D. thesis, McGill University, Montreal.