Circular Basin

UNDER CONSTRUCTION

Problem

Dupont (2001) presented an analytical solution for a closed circular domain on an f-plane with a linear bottom friction. The governing equations are \begin{equation} \tag{1}

 \frac{\partial H U}{\partial x} + \frac{\partial H V}{\partial y} = 0

\end{equation}

\begin{equation} \tag{2}

-f_c V + g \frac{\partial \eta}{\partial x} =  \kappa U + \frac{W y}{R H}

\end{equation}

\begin{equation} \tag{3}

Failed to parse (syntax error): {\displaystyle  f_c U + g \frac{\partial \eta}{\partial y} =  \kappa V \end{equation}  where <math>U}
 and $V$ are the depth-averaged current velocities in the $x$ and $y$ directions respectively, $g$ is the gravitational constant, $\eta$ is the water surface elevation with respect to mean sea level, $\kappa$ is a linear bottom friction coefficient, $R$ is the radius of the domain, $H$ is the water depth, and $W$ is a constant equal to the gradient of the wind forcing.

Solution

The analytical solution for water surface elevation solution is given by

$\eta ={\begin{cases}{\frac {W{{r}^{2}}\sin 2\theta }{4gHR}},&{\mbox{if }}f_{c}=0\\{\frac {W{{f}_{c}}}{RgH\kappa }}\left[{\frac {{R}^{2}}{8}}+{\frac {{r}^{2}}{4}}\left({\frac {\kappa }{{f}_{c}}}\sin 2\theta -1\right)\right],&{\mbox{if }}f_{c}\neq 0\\\end{cases}}$

(1)

The current velocities are independent of the Coriolis parameter and are given by

$U={\frac {Wy}{2RH\kappa }}$

(3)

$V=-{\frac {Wx}{2RH\kappa }}$

(4)

Setup

Figure 1. Computational grid.

The model is run to steady state from zero current and water level initial conditions with $W=10^{-}4{\text{m}}^{2}{\text{s}}^{-2}$, $\kappa =10^{-3}{\text{s}}^{-1}$ , and $f_{c}=0$. Table 1 shows the general settings used for CMS-Flow. Figure 1 shows the computational grid with 5 levels of refinement from 2000 m to 125 m.

Table 1. General Settings for Wind-driven flow in a circular basin

Parameter	Value
Time step	3600 s
Simulation Duration	72 hrs
Ramp Period	24 hrs
Initial Water Depth	100 m
Mixing Terms	Off
Wall Friction	Off
Linear Bottom Friction Coefficient	0.001

Results

No Coriolis

Figure 1. Analytical current velocities and water levels for the case without Coriolis.

Figure 2. Calculated current velocities and water levels for the case without Coriolis.

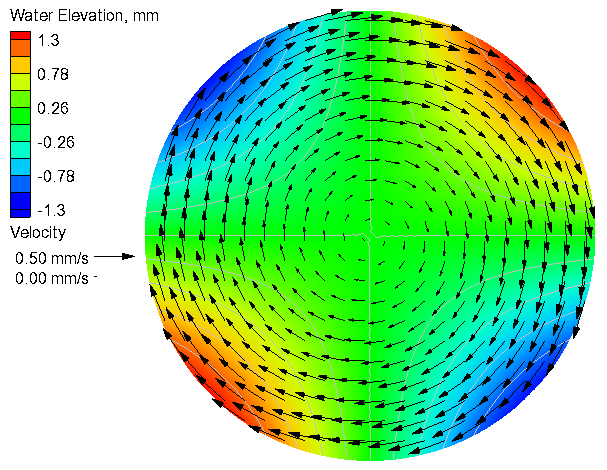
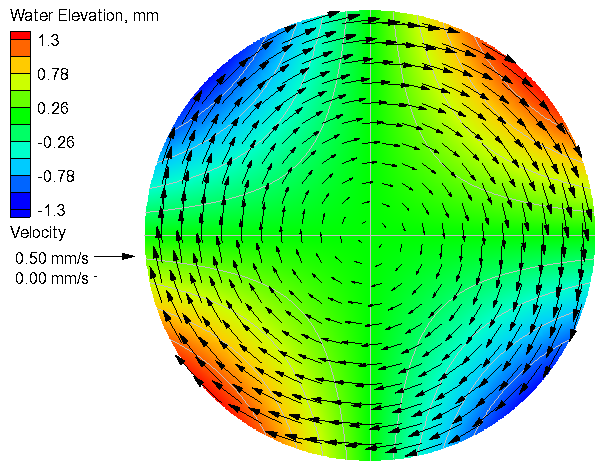
Table 2. Goodness of fit statistics for the current velocity and water level

Variable	NRMSE, %	NMAE, %	R^2	Bias
U-Velocity	2.52	0.37	0.999	-8.5e-8 m/s
V-Velocity	2.53	0.38	0.999	7.26e-8 m/s
Water Level	0.03	0.02	0.999	-3.5e-7 m

• For a definition of the goodness of fit statistics see Goodness of fit statistics.

With Coriolis

Figure 3. Analytical current velocities and water levels for the case with Coriolis.

Figure 4. Calculated current velocities and water levels for the case with Coriolis.

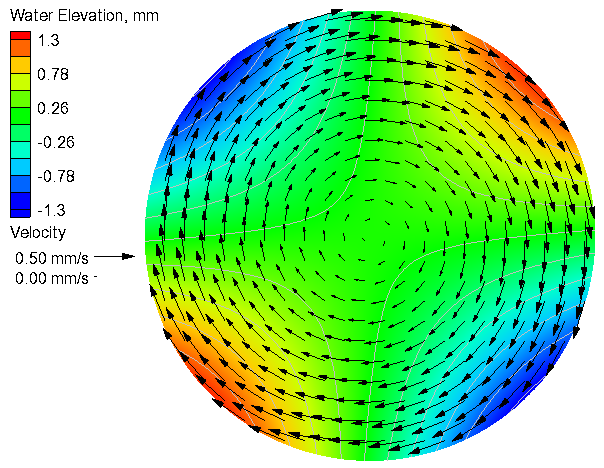
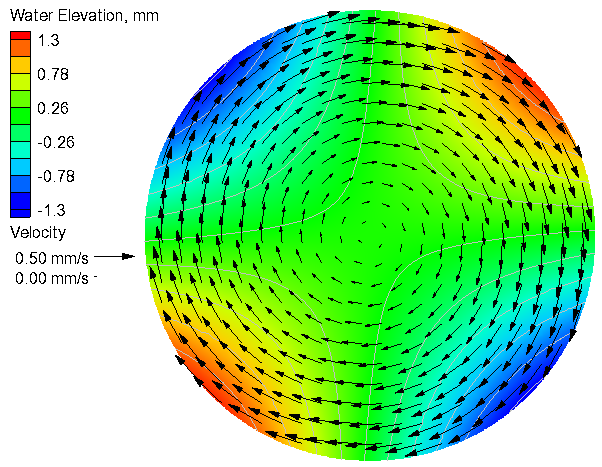
Table 3. Goodness of fit statistics for the current velocity and water level

Variable	RRMSE, %	RMAE, %	R^2	Bias
U-Velocity	2.53	0.37	0.999	-8.5e-8 m/s
V-Velocity	2.56	0.37	0.999	6.5e-8 m/s
Water Level	0.03	0.02	0.999	-3.0e-7 m

References

• Dupont, F., 2001. Comparison of numerical methods for modelling ocean circulation in basins with irregular coasts. Ph.D. thesis, McGill University, Montreal.

---

Test Cases

Documentation Portal