Long-wave Runup
UNDER CONSTRUCTION
Overview
The goal of this verification test to asses the model performance in simulating nonlinear runup/rundown over a plane slopt. Carrier et al. (2003) presented an analytical solution to the nonlinear shallow water equations over a plane slope for several initial wave forms. Here the analytical solution for a the initial wave form given by leading-depression N-wave is used.
Initial Condition
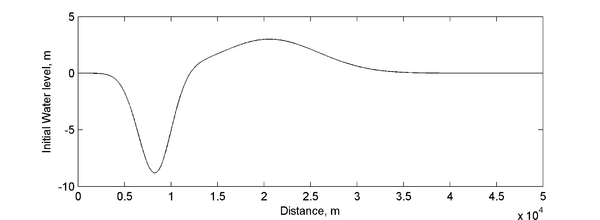
The bed has a constant slope of 1/10 with the initial shoreline located at x=0. Figure 1 shows the initial water level (is given by a leading depression N-wave (characteristic of the waves caused by submarine landslides). The initial current velocity is equal to zero everywhere.
Model Setup
The computational grid has a 3 m resolution for x<300 and increases to 10 m with an aspect ratio of 1.05. The general model parameters used in the simulation are shown in Table 1.
Table 1. Model Parameters
| Parameter | Value |
|---|---|
| Time step | 0.1 s |
| Ramp period | 0.0 s |
| Drying depth | 0.01 m |
| Wall friction | Off |
| Mixing terms | Off |
| Manning's coefficient | 0.0 |
Results
Figure 2 shows a comparison of computed and analytical water surface elevations near the shoreline at 4 different time steps. The goodness of fit statistics are shown in Table 2.
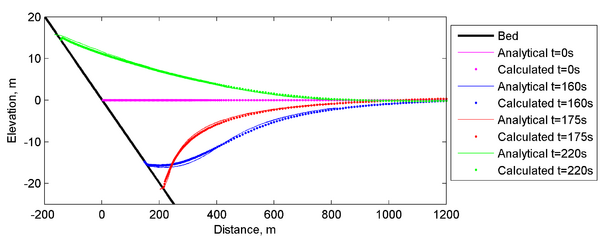
Table 2. Goodness of Fit Statistics
| Time, s | RRMSE, % | RMAE, % | R^2 | Bias, m |
|---|---|---|---|---|
| 160 | 3.7 | 3.8 | 0.999 | -0.012 |
| 175 | 6.5 | 5.9 | 0.997 | -0.113 |
| 220 | 4.6 | 5.4 | 1.000 | -0.066 |
- For a definition of the goodness of fit statistics see Goodness of fit statistics.
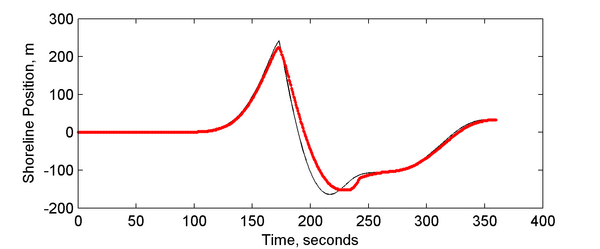
A comparison of the calculated and analytical shoreline position are shown in Figure 3.
References
- Carrier, G., Wu, T.T., and Yeh, H. (2003). "Tsunami runup and draw-down on a plane beach", Journal of Fluid Mechanics, 475, 79-99.